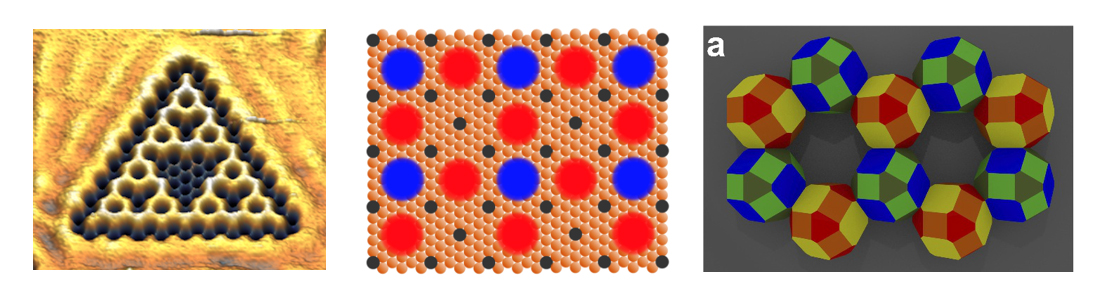
Quantum simulations in electronic systems:  Lieb, Sierpinski, Kagome, Kekule, graphene

Sixty years ago, Feynman proposed that we could build matter in a bottom-up approach to simulate model Hamiltonians that are supposed to describe complex materials, or even to build metamaterials. Although the field of quantum simulators has been flourishing in ultracold atoms for about 20 years, and in photonics for about 15 years, almost nothing was done involving electrons. We are actively contributing to the field. The experimental platform is based on the pioneering works by the group of Don Eigler (Almaden), who created a quantum corral by patterning Fe atoms in a circle on Cu(111) and showed quasi-particle interference. Some years later, Hari Manoharan’s group used the same technique to pattern a triangular lattice of CO molecules on Cu(111). The CO molecules act as potential barriers and generate a honeycomb lattice for the electrons. However, one can only place the COs on top of the copper atoms, and those have a triangular geometry. Hence, if this approach would serve to build only triangular or honeycomb lattices, it would not be very useful. 

First, we have shown that one can use this platform to build also square and Lieb lattices [1]. Then, we have shown how to control the orbital (px and py) degrees of freedom, in real space and in energy. By appropriately engineering the lattice, we were able to lift the degeneracy of the in-plane p-orbitals [2]. Next, we have shown that we can not only control the geometry of the lattice, but also its dimensionality. To this aim, we created three generations of a Sierpinski fractal, and have theoretically and experimentally proven that the electronic wavefunction lives in 1.58 dimension [3]. This work has attracted great attention of the media; it was highlighted in Physics Today and in many European scientific journals, and a Youtube movie made by Seeker has already more than 700 thousand views. Then, we have provided the first experimental realization of a (dipolar) higher-order topological insulator (HOTI) with electrons [4], by creating a breathing kagome lattice in the trivial and topological phases. Moreover, we investigated how the existence of edge modes depends on the sample termination in topological crystalline insulators by realising different Kekule lattices [5]. Finally, we have shown how to engineer flat p-bands in graphene-like structures, which are well separated from the s-bands [6]. 

In a different kind of setup, we have shown how topological edge states arise in self-assembled 2D honeycomb lattices made of CdSe semiconducting nanocrystals [7]. Theoretical studies using a first-principle technique and a 16-band tight-binding model have predicted a quantum spin Hall effect in the valence band, for holes. We have also envisaged the possibility to realize the same kind of lattices using HgTe, and have shown theoretically that a fascinating system, with Dirac cones, flat bands, and a room-temperature quantum spin Hall effect can be achieved upon doping the nanocrystals with a few electrons [8].

[1] Experimental realization and characterization of an electronic Lieb lattice,

M. R. Slot, T. S. Gardenier, P. H. Jacobse, G. C. P. van Miert, S. N. Kempkes, S. J. M. Zevenhuizen, C. Morais Smith, D. Vanmaekelbergh, I. Swart,

Nature Physics 13, 672 (2017). 

[2] p-band engineering in artificial electronic lattices”, 

M. R. Slot, T. S. Gardenier, P. H. Jacobse, G. C. P. van Miert, S. N. Kempkes, S. J. M. Zevenhuizen, C. Morais Smith, D. Vanmaekelbergh, I. Swart,

Phys. Rev. X 9, 011009 (2019). 

[3] Design and characterization of electronic fractals, 

S. N. Kempkes, M. R. Slot,   S. E. Freeney, S.J.M. Zevenhuizen,   D. Vanmaekelbergh, I. Swart, and C. Morais Smith,  

Nature Physics 15, 127 (2019).

[4] Robust zero-energy modes in an electronic higher-order topological insulator: the dimerized Kagome lattice

S. N. Kempkes, M. R. Slot,  J.J. van den Broeke, P. Capiod, W. Benalcazar, D. Vanmaekelbergh, D. Bercioux, I. Swart, and C. Morais Smith

Nature Materials 18, 1292 (2019).

[5] Edge-dependent topology in Kekule lattices

S. E. Freeney, J.J. van den Broeke, A.J.J. Harsveld van der Veen,  I. Swart, and C. Morais Smith,
ArXiv: 1906.09051, under peer review in Phys. Rev. Lett. (2020).

 [6] p-Orbital flat band and Dirac cone in the electronic honeycomb lattice

 T. S. Gardenier, J.J. van den Broeke, J. Moes, I. Swart, C. Delerue, M. R. Slot, C. Morais Smith, and D. Vanmaekelbergh
ArXiv: 2004.03158, under peer review in Phys. Rev. Lett. (2020). 

[7] Topological states in multi-orbital HgTe honeycomb lattices,

W. Beugeling, E. Kalesaki, C. Delerue, Y. -M. Niquet, D. Vanmaekelbergh, C. Morais Smith,

Nature Commun. 6, 6316 (2015).

[8] Dirac Cones, Topological Edge States, and Nontrivial Flat Bands in Two-Dimensional Semiconductors with a Honeycomb Nanogeometry,

E. Kalesaki, C. Delerue, C. Morais Smith, W. Beugeling, G. Allan, D. Vanmaekelbergh,

Physical Review X 4, 011010 (2014).