Thermodynamics of topological insulators

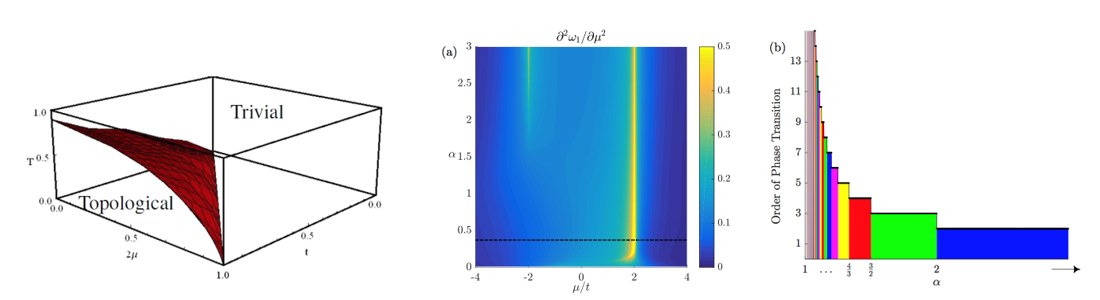
Topological insulators are states of matter distinguished by the presence of symmetry protected metallic boundary states. These edge modes have been characterised in terms of transport and spectroscopic measurements, but a thermodynamic description has been lacking. Recently, we have shown that using Hill thermodynamics, it is possible to separate the edge and bulk thermodynamic potential, and describe the topological phase transition using thermodynamic observables, such as heat capacity or density of states [1]. Subsequently, we extended this approach to different topological models in various dimensions (the Kitaev chain and Su-Schrieffer-Heeger model in one dimension, the Kane-Mele model in two dimensions and the Bernevig-Hughes-Zhang model in two and three dimensions) at zero temperature. Surprisingly, all models exhibit the same universal behavior in the order of the topological-phase transition, depending on the dimension. A D-dimensional model has D-th order phase transition at the edge and (D + 1) th order in the bulk [2]. These results can be understood through the Josephson hyperscaling relation, given in terms of dynamical exponents [3]. This thermodynamic description allows one to calculate the topological phase diagram at finite temperatures, which shows a very good agreement with the one calculated from the Uhlmann phase [2] (see figure). More recently, we investigated also Kondo topological insulators, such as SmB6, and have that they belong to the same universality class as the other topological insulators. We have also shown that the upturn in the heat capacity of SmB6 is not due to the edge contribution [3], as originally conjecture. In addition, we investigated the long-range Kitaev chain [4], and found that the order of the bulk phase transition depends on the range of the interaction: for short-range, it is second order, but it becomes infinite order, in a staircase way, for long-range interactions (see figure). Our work reveals unexpected universalities and opens the path to a thermodynamic description of systems with a non-local order parameter. More recently, we have investigated a higher-order topological insulator using the thermodynamic formalism, and have shown that although the usual thermodynamic potential is not able to capture all the phase transitions, a so-called Wannier grand-potential can do so [5]. Finally, we established the connection to heat engines by using the thermodynamic formalism for a Kitaev chain [6].

[1] Thermodynamic signatures of edge states in topological insulators,

A. Quelle, E. Cobanera, and C. Morais Smith, 

Phys. Rev. B 94, 075133 (2016). 

[2] Universalities of thermodynamic signatures in topological phases,

S. N. Kempkes, A. Quelle, and C. Morais Smith,

Nature Scientific Reports 6, 38530 (2016).

[3] Thermodynamic study of topological Kondo insulators,

J. J. van den Broeke, S. N. Kempkes, A. Quelle, and C. Morais Smith,

arXiv:1803.03553.

[4] Staircase to higher-order topological phase transitions,

P. Cats, A. Quelle, O. Viyuela, M. A. Martin-Delgado, and C. Morais Smith,

Phys. Rev. B 97, 121106 (R) (2018). 

[5] Thermodynamics of a higher-order topological insulator, 

R. Arouca, S. N. Kempkes, and C. Morais Smith,

Phys. Rev. Research 2, 023097 (2020)

[6] Topological friction in a Kitaev chain heat engine 

E. Yun, M. Fadaie, O. E. Mustecaplioglu, and C. Morais Smith,

ArXiv: 2003.08836, under peer review in Phys. Rev. B (2020)