Out of equilibrium systems: Floquet theory for ultracold-atoms and condensed-matter systems

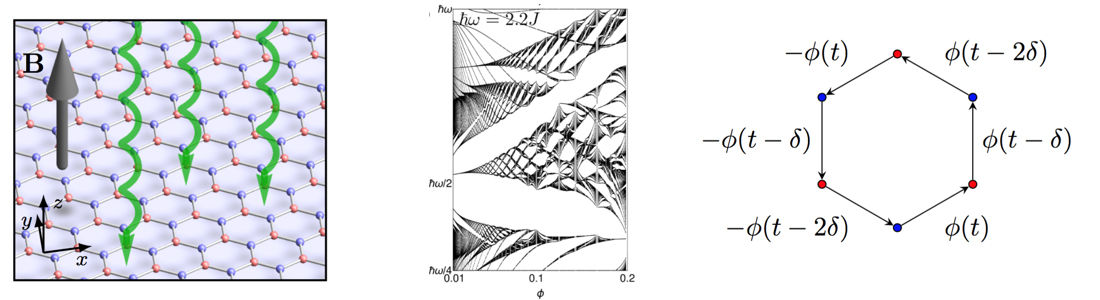
Systems under the influence of time-periodic perturbation, the so-called Floquet systems, have attracted great interest in condensed matter and cold atom systems. They have been theoretically and experimentally investigated in three different regimes: When the driving frequency is much larger than the bandwidth, but much smaller than the bandgap, the system constituents cannot follow the perturbation, and the system remains at quasi-equilibrium with simply renormalised lattice parameters. The second regime, when the shaking frequency is comparable to the gap, has been recently investigated in cold atoms, but it remains unexplored in the context of condensed matter. We have mostly studied the third regime, when the frequency is comparable to the bandwidth. For a circularly shaken honeycomb optical lattice, the transition between the first and third regimes can be understood in terms of band inversion because with decreasing frequency, the different Floquet bands start to overlap. We investigated the influence of the time-dependent driving on the quantum Hall states generated by a perpendicular magnetic field and found that the butterfly-wing shape of the spectrum can be analytically understood in terms of avoided crossings in the Landau-level regime [1]. In the case of topological insulators, the driving can lead to very interesting phenomena and novel phases, which do not have a static counterpart. A curious example is a phase that hosts protected edge states but has Chern number zero [2]. We also studied the competition between a quantum spin Hall effect, generated by an intrinsic spin-orbit coupling, and the dynamical Haldane phase, driven by circular shaking [3,4]. In the first regime, when the driving frequency is much larger than the bandwidth or the Hubbard interaction, but smaller than the bandgap, we investigated a 1D lattice in the presence of a driven Feshbach resonance (dc + ac components). We found that the effective quasi-equilibrium model for fermions than corresponds a correlated-hopping model, conjectured to exhibit eta-pairing superconductivity [5]. In the 2D case, for a honeycomb lattice, the usual linear shaking in this high-frequency regime may lead to the merging or alignment of the Dirac cones, depending on the direction of the shaking. When the Dirac cones merge, a semi-metal/insulator transition takes place [6].