Marta Pieropan
Mathematical Institute
Email: m.pieropan@uu.nl
|
 |
|
Research interests
algebraic geometry and number theory, Diophantine geometry, analytic number theory
algebraic geometry over nonclosed fields
rational points, Campana points, integral points
geometry of numbers and o-minimality, hyperbola method, circle method
number fields, global fields
Cox rings
Fano varieties, rationally connected varieties, toric varieties
arithmetic applications of birational geometry
arithmetic applications of logarithmic geometry
Overview
My research area, arithmetic geometry, is located at the intersection of algebraic geometry and number theory. Most of my work can be classified as Diophantine geometry, an area of arithmetic geometry that studies integer and rational solutions to polynomial equations using ideas and techniques from algebraic geometry. I am particularly committed to investigating the principle that geometry determines arithmetic in the case of Fano and rationally connected varieties, especially in relation to birational geometry and logarithmic geometry.
Diophantine problems
Many Diophantine problems arise from basic questions about the shape of the sets of integer or rational solutions to systems of polynomial equations in several variables. I am interested in fundamental quantitative questions like: Is the set of solutions empty? Is it finite? If so, how many solutions are there? If the set of solutions is infinite, one can ask questions about the size of the solutions or their arithmetic complexity, which are measured via height functions. One classical example is the Weil height, which is a function $H: \mathbb Q^n \to \mathbb R_{\geq0}$ defined as follows. For a point $x$ in $\mathbb Q^n$, represented by $\left(\frac {a_1} {a_0}, \dots, \frac {a_n} {a_0}\right)$ for suitable integers $a_0,\dots,a_n$, the Weil height is given by \[ H(x) = \frac { \max\{ | a_0|, \dots, |a_n|\} } { \gcd(a_0,\dots,a_n)}. \] We observe that the set of points of $\mathbb Q^n$ of height at most $B$ is finite for every real number $B$ (see the figure below for an example). Hence, given a system of polynomial equations it makes sense to count the number of solutions of height at most $B$, and some of the quantitative questions asked above can be answered by studying the asymptotic behavior of such cardinalities for $B\to +\infty$.
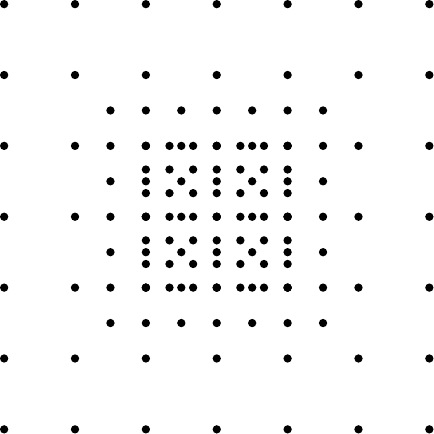
Points in $\mathbb Q^2$ of Weil height at most $3$.
Rational points on algebraic varieties
Diophantine problems can be studied by means of algebraic geometry. Indeed, systems of polynomial equations in many variables define algebraic varieties, which are the basic objects of study in algebraic geometry. Under this correspondence, solutions over a field are called rational points over that field. The set of rational points usually varies under extension of the base field. For example, the algebraic variety defined by the equation $x^2+y^2=3$ has empty set of rational points over the rational numbers (because the equation has no solutions in $\mathbb Q$), but nonempty set of rational points over the real numbers, as $x=\sqrt 3$, $y=0$ is a solution.
In this setting, the height functions are $\mathbb R$-valued functions on the set of rational points associated to line bundles on the algebraic variety. For example, the Weil height described above is a height function on the projective space $\mathbb P^n$ over $\mathbb Q$ associated to the line bundle $\mathcal O(1)$. By their very nature, height functions translate problems about rational points into questions about numbers (the heights), and provide a fundamental tool that relates geometric properties to number theoretic ones. For example, they have been used to prove major theorems in Diophantine geometry like the Mordell–Weil Theorem and Faltings' proof of the Mordell–Lang conjecture.
Geometry determines arithmetic
The goal of Diophantine geometry is to describe the sets of solutions of Diophantine equations in terms of the geometric properties and invariants of the associated algebraic varieties. From the point of view of rational points over $\mathbb Q$, smooth algebraic varieties can be divided into three classes according to the positivity of their canonical bundle. Varieties with very positive canonical bundle are called of general type. For those, Lang conjectured that the set of rational points over a number field is contained in a proper subvariety. On varieties of intermediate type (for example, abelian varieties) the set of rational points is dense in some cases, not dense in others. Among varieties with nonpositive canonical bundle there are Fano varieties, rational varieties and rationally connected varieties. For those the set of rational points is expected to be dense over a finite extension of $\mathbb Q$ (potential density). I investigate how the geometry of this last class of varieties affects their sets of rational points over nonclosed fields of characteristic zero.
Rationally connected and Fano varieties
In characteristic zero, Fano varieties are the fundamental examples of rationally connected varieties. Indeed, using the Minimal Model Program all rationally connected varieties can be built out of terminal (i.e., mildly singular) Fano varieties (up to birational equivalence). Moreover, Fano varieties enjoy two finiteness properties that in general don't hold for (smooth) rationally connected varieties and can be exploited to study their arithmetic properties:
Boundedness: There are only finitely many deformation families of terminal Fano varieties in each dimension.
MDS: Terminal Fano varieties are Mori Dream Spaces, which means that they have finitely generated Cox rings.
The first property results in the explicit classification of Fano varieties in low dimension. The second property enables concrete descriptions of the set of rational points over number fields as lattice points on an auxiliary space (a torsor). I use both properties to study the arithmetic of rational points on Fano and rationally connected varieties.
One of the fascinating facts about Fano varieties is that though there are few of them because of boundedness, when it comes to their arithmetic properties there is no established method to study all of them at the same time. Typically, one needs to develop ad hoc techniques for each variety. The goal of my research is to experiment and develop new methods toward a unified approach.
Distribution of rational points on Fano varieties: Manin's conjecture
The distribution of rational points on a variety is measured by height functions, associated to certain line bundles, that satisfy the Northcott property (that is, the number of rational points of height at most $B$ is finite for every $B>0$). For smooth Fano varieties with a dense set of rational points, Manin conjectured that on a suitable subset of the variety the number of points of anticanonical height bounded above by $B$ grows like \begin{equation*} CB(\log B)^{r-1}\quad\text{as}\quad B\to+\infty, \end{equation*} where $C$ is a positive constant and $r$ is the rank of the Picard group of the variety. This asymptotic has been verified for some families of varieties and many single cases, but the conjecture is still widely open. The main reasons are the lack of a unified approach that could treat all Fano varieties at once and the fact that the classification of Fano varieties in high dimension is far from complete.
One of the methods to verify Manin's conjecture for a given variety is called universal (or split) torsor method and consists in: a parameterization step via universal (or split) torsors and Cox rings, and a counting step via ad hoc lattice point counting techniques. Unlike other approaches, the split torsor method in principle could be applied to every Fano variety, but it has not been developed in sufficient generality yet. Part of my work [2, 4] consisted in making the parameterization step systematic, whereas previously the parameterizations were obtained by ad hoc constructions case by case. The counting step is still far from being systematic.
The split torsor method is designed to work well over $\mathbb Q$, as severe additional difficulties of a number theoretic nature appear over arbitrary number fields. In particular, the counting step becomes the problem of counting orbits under the action of a (possibly infinite) group on a lattice. As a consequence, the literature on the split torsor method over arbitrary number fields is still limited. My contributions [1, 2, 4] are part of the first significant steps towards overcoming these problems.
Cox rings. Cox rings are certain graded rings that have been constructed and studied for varieties over algebraically closed fields in the context of birational geometry. In [3] we study Cox rings over arbitrary fields via a new axiomatic approach that leads to classification and existence results that agree with the theory of torsors under quasitori and provides the theoretical basis for arithmetic applications of finitely generated Cox rings to the parameterization of rational points.
Parameterization. To reduce the verification of Manin's conjecture to a lattice point counting problem, one needs integral models of universal (or split) torsors. In [2, 4] we give an explicit construction procedure of such models over maximal orders of arbitrary number fields, and obtain computational criteria to determine whether they satisfy important properties like smoothness, projectivity, etc.
Counting step. In [1] I extend work of Salberger to imaginary quadratic fields. It is the first application of the universal torsor method to a family of varieties (not just a single variety) over number fields beyond $\mathbb Q$. In [2] we use universal torsors to verify Manin's conjecture for a certain singular del Pezzo surface of degree 4 over arbitrary number fields. The originality of the proof lies in the new approach to lattice point counting via o-minimality. In [4] we use split torsors to verify Manin's conjecture for all the Galois twists of the same singular del Pezzo surface of degree 4. The main new difficulty is due to the existence of primes of bad reduction.
Arithmetic of Campana orbifolds
In 2004 Campana introduced the notion of orbifold structure on the base of a fibration. It consists of a $\mathbb Q$-divisor $\Delta$ with coefficients in $]0,1]$ that encodes the multiplicities of the pullbacks of prime divisors. He used it to decompose projective varieties into fibrations whose building pieces are either of general type or special (e.g., rationally connected or Calabi-Yau). Besides providing a birational classification alternative to the Minimal Model Program, orbifolds interpolate between projective ($\Delta=0$) and quasi-projective varieties (coefficients of $\Delta$ all equal to 1). Campana points are rational points on orbifolds with intersection multiplicity with the boundary divisor prescribed by the coefficients of $\Delta$. Hence, Campana points on an orbifold over a number field interpolate between rational points and integral points with respect to $\Delta$. The study of raises very interesting and difficult number theoretic questions. For example, Campana's generalization of Lang's conjecture to orbifold curves is related to the $abc$-conjecture. Also counting Campana points of bounded height can translate into challenging problems like, for example, estimating the number of positive squareful integers $x,y\leq B$ such that $x+y$ is squareful, which has been open for more than ten years.
A Manin-type conjecture for Campana points. Besides the vast literature on Manin's conjecture for rational points, there is also an interest in asymptotics for integral points. The interaction between geometry and arithmetic is more subtle in this case, and has so far prevented the formulation of a conjecture. In [7] we look at the intermediate notion of Campana points. We formulate an analog to Manin's conjecture for orbifolds of Fano type and we prove it for compactifications of vector groups. The distribution of rational points and integral points on compactifications of vector groups has been determined by Chambert-Loir and Tschinkel. Our work agrees with the concept that Campana points interpolate between rational and integral points.
Hyperbola method on toric varieties. In collaboration with D. Schindler [8], I investigate the distribution of Campana points of bounded height on toric varieties with boundary divisor invariant under the torus action. We use a parameterization of the set of Campana points via universal torsors and we develop a version of the hyperbola method to count points of bounded height on toric varieties. Using this method, we show that the conjecture in [7] holds for many split toric varieties over $\mathbb Q$ equipped with the log-anticanonical height.
Rational points on rationally connected varieties over $C_1$ fields: $C_1$-conjecture
A $C_1$ field is by definition a field $k$ such that every homogeneous polynomial of degree $d\leq n$ in $n+1$ variables has a nontrivial solution in $k$ (equivalently, every hypersurface of degree $d\leq n$ in $\mathbb P^n$ has a rational point over $k$). A smooth hypersurface in $\mathbb P^n$ is Fano if and only if it is rationally connected if and only if it has degree $d\leq n$. The $C_1$-conjecture, due to Lang (2000), states that proper smooth rationally connected varieties over a $C_1$ field have a rational point. The conjecture has been proven for the following $C_1$ fields: finite fields by Esnault, function fields of curves over algebraically closed fields by Graber, Harris, de Jong and Starr. The case that remains open is that of the maximal unramified extension of a non-archimedean local field of characteristic zero (e.g., $\mathbb Q_p^{\mathrm{nr}}$).
Reduction to Fano. In [6] I use birational geometry over nonclosed fields to reduce the $C_1$-conjecture in characteristic zero to the problem of finding rational points on Fano varieties with terminal singularities. By boundedness this means that the problem is reduced to finding rational points on finitely many families of Fano varieties in each dimension. As a consequence, with a suitable application of the transfer principle from model theory, I show that rationally connected varieties of fixed dimension have rational points over $\mathbb Q_p^{\mathrm {nr}}$ for all sufficiently large primes $p$, thereby improving upon work of Duesler and Knecht. Using the same method I also show that in characteristic zero there is a uniform upper bound, depending only on the dimension, for the degree of the minimal extension of the base field where a rationally connected variety acquires a rational point.
Evidence in characteristic zero. The $C_1$-conjecture is known to hold in dimension $\leq 2$. In [6] I use the current classification of terminal Fano varieties (which is still incomplete) to provide evidence that supports the conjecture in dimension $\geq 3$. In particular, I show that the following rationally connected varieties have points over $C_1$ fields: toric varieties, complete intersections in products of projective spaces, Gorenstein Fano varieties of dimension $n$ and index $\geq n-1$, and a number of Fano threefolds of index 1. In order to use the classification results that hold over algebraically closed fields, I also study the Galois descent of complete intersections via Cox rings [5].
[1] M. Pieropan, Imaginary quadratic points on toric varieties via universal torsors, Manuscripta Math. 150, no. 3, pp. 415-439, 2016. DOI:10.1007/s00229-015-0817-8 Preprint: arXiv:1505.05789
[2] C. Frei and M. Pieropan, O-minimality on twisted universal torsors and Manin's conjecture over number fields, Ann. Sci. Éc. Norm. Supér. (4) 49, no. 4, pp. 757-811, 2016. DOI: 10.24033/asens.2295 Preprint: arXiv:1312.6603
[3] U. Derenthal and M. Pieropan, Cox rings over nonclosed fields, J. Lond. Math. Soc. 99, no.2, pp.447-476, 2019. DOI: 10.1112/jlms.12178 Preprint: arXiv:1408.5358
[4] U. Derenthal and M. Pieropan, The split torsor method for Manin's conjecture, Trans. Amer. Math. Soc., to appear, DOI: 10.1090/tran/8133 Preprint: arXiv:1907.09431
[5] M. Pieropan, On Galois descent of complete intersections, Math. Res. Lett. 28, no. 4, pp. 1243–1254, 2021. DOI: 10.4310/MRL.2021.v28.n4.a12 Preprint: arXiv:1905.00227
[6] M. Pieropan, On rationally connected varieties over C_1 fields of characteristic 0, Algebra Number Theory, to appear. Preprint: arXiv:1905.02227
[7] M. Pieropan, A. Smeets, S. Tanimoto and A. Várilly-Alvarado, Campana points of bounded height on vector group compactifications, Proc. Lond. Math. Soc. 123, no. 3, pp. 57–101, 2021. DOI: 10.1112/plms.12391 Preprint: arXiv:1908.10263
[8] M. Pieropan and D. Schindler, Hyperbola method on toric varieties, Preprint: arXiv:2001.09815